First of all, let’s get one thing straight: I am not a mathematician.
Growing up, I loved art and music and I avoided math whenever I could. But eventually I realized I was really missing out on something good. Math is cool. It’s beautiful, and surprising, and much, much more interesting than I ever suspected.
Geometric Series
The Lion’s Share is a book about what happens when you cut things in half and when you double them. Halving and doubling are examples of what mathematicians call a geometric series or a geometric progression.
It sounds complicated, but it’s not. To make a geometric series, just start with a number. Let’s use the number one:
1
Now, multiply it by something (called the common ratio) to get the next number in the sequence. In The Lion’s Share, the animals all promise to bake twice as many cakes as the next, so we’ll use 2 for our common ratio:
1 x 2 = 2
Now, we multiply our answer (2) by the common ratio (also 2) to get the next number:
2 x 2 = 4
If we keep doing this, we’re making a geometric series:
| 1 | x 2 = | 2 | x 2 = | 4 | x 2 = | 8 | x 2 = | 16 | x 2 = | 32… |
We can do this for as long as we want. The series never ends; it just keeps getting bigger and bigger. A lot bigger. This is why the elephant ended up baking so many cakes, even though there were just a few animals at dinner.
Changing the ratio
What happens if we use a number larger than 2 for the common ratio? How about 3?
| 1 | x 3 = | 3 | x 3 = | 9 | x 3 = | 27 | x 3 = | 81 | x 3 = | 243… |
Or 4?
| 1 | x 4 = | 4 | x 4 = | 16 | x 4 = | 64 | x 4 = | 256 | x 4 = | 1,024… |
As you can see, making the ratio just a little bit bigger can make huge changes in the series after just a few steps. It’s a good thing the animals didn’t offer to triple or quadruple the number of cakes they would bake.
Getting smaller
Now let’s try this the other way. What happens if we make the ratio smaller?
Let’s try a value of 1:
| 1 | x 1 = | 1 | x 1 = | 1 | x 1 = | 1 | x 1 = | 1 | x 1 = | 1… |
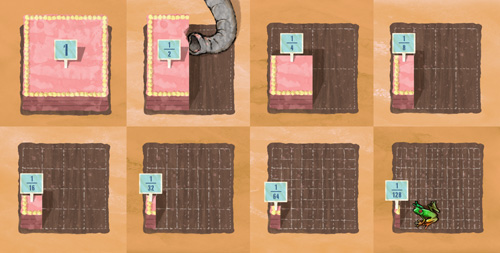
Answer? Nothing happens. Boring. But how about if we go even smaller? Here’s what it looks like with a ratio of 1/2:
| 1 | x 1/2= | 1/2 | x 1/2 = | 1/4 | x 1/2 = | 1/8 | x 1/2 = | 1/16 | x 1/2 = | 1/32… |
Now we’re getting somewhere. As you can see, the numbers get small really quickly. This is what’s happening when the animals cut the cake at the beginning of the book. It doesn’t take too many cuts before the remaining slice is too small even for the ant.
Number systems
Our whole number system is based on a geometric series. We have a ones place, a tens place, a hundreds place, and so on…
Looked at another way (with your head turned ninety degrees) this is just a geometric series with a common ratio of 10:
| 1 | x 10 = | 10 | x 10 = | 100 | x 10 = | 1000 | x 10 = | 10000.. |
Computers use the binary system, which means they only use two different digits, zero and one. Instead of ones, tens, and hundreds places, they use ones, twos, and fours places. Their number system looks like this:
Remember this series? It’s the one we started with, and it has a common ratio of 2:
| 1 | x 2 = | 2 | x 2 = | 4 | x 2 = | 8 | x 2 = | 16 | x 2 = | 32… |
These numbers turn up a lot in the computer field. If you buy an iPod, for example, you might find that it’s sold in 2GB, 4GB, and 8GB models. That’s because, to a computer, these are nice, round numbers, in the same way that 10, 100, and 1000 are to us decimal-loving humans.
Once you start looking for it, the geometric series turns up everywhere. It’s used to carbon-date archeological finds, figure bank loans, and even map the way viruses multiply and family trees grow.
Pretty cool, for something so simple.